Mathematical Astronomy in Babylon
March 10, 2016
My
science education started with
children's science books. One of these was "
The World of Science," and another was the "
Golden Book of Science."[1] The full title of the latter book was, "The Golden Book of Science for Boys and Girls." The
author of that book was a
woman, Bertha Morris Parker, and this probably explains such an early excursion into
gender equality in the sciences. The premier children's
mathematics book of my generation was
Irving Adler's, "The Giant Golden Book of Mathematics," Illustrated by Lowell Hess (
Golden Press,
New York, 1960).[2]

The Golden Book of Science for Boys and Girls by Bertha Morris Parker (1956).
This profusely illustrated book was an inspiration for many young scientists.
It's interesting how far science has advanced in the sixty years since publication of this book.
(Scan of the cover of my copy.)[1])
![]()
One frequent topic in children's books at that time was the
Seven Wonders of the Ancient World, as listed below.
While the existence of the Giza Pyramid is quite evident, and the existence of all but one of the other "wonders" is verifiable, the location of the Hanging Gardens of Babylon has never been established. They were thought to have been built by
King Nebuchadnezzar II in 600
BC in the ancient city of
Babylon, located in today's
Iraq. The Hanging Gardens might have been purely
mythical, or they may have just described a known
garden built by the
Assyrian king,
Sennacherib, in nearby
Nineveh.
.jpg)
The Hanging Gardens of Babylon, as painted in 1886 by Ferdinand Knab (1834-1902) as part of his "Seven Wonders of the Ancient World" series.
(Via Wikimedia Commons.)
Although we question Babylonian excellence in
gardening, there's no question that Babylonian mathematics was advanced for its time. One
quirk of Babylonian mathematics was its
number system, which was
sexagesimal; that is, it was a
base-60 system. This number system, probably inherited from the
Sumerians, had some
logic behind it. Sixty is a
highly composite number, having as factors, 1, 2, 3, 4, 5, 6, 10, 12, 15, 20, 30, and 60. A Babylonian
cook would have an easy time
scaling recipes.

Some representative Babylonian numbers, illustrating how quantities were inscribed by a simple wedge-tipped stylus into clay tablets. fractional numbers, as given in modern notation, have the form a; b, c, d, which has the decimal equivalent a + (b/60) + (c/3600) + (d/603), etc. (Modified Wikimedia Commons image by Josell7.)
This sexagesimal number system might be the reason why we have 360
degrees in a
circle. The Babylonians used an inscribed
hexagon to divide a circle, and each sixth of that circle was further divided by 60, resulting in 360 degrees.
Like most other ancient people, the
Babylonians were avid astronomers, and they recorded their
astronomical observations on
clay tablets. As an example, the
Venus tablet of Ammisaduqa, which dates to about 1650 BC, records the risings and setting times of
Venus over a period of 21 years. There's even an observation of
Halley's comet in 164 BC (see figure).

Perhaps it was practice in deciphering the hen-scratching of students that enabled the deciphering of this tablet.
A Babylonian tablet, now at the British Museum in London, recording the appearance of Halley's comet in 164 BC.
(Photograph by Linguica, via Wikimedia Commons.)
In a recent article in Science,
Mathieu Ossendrijver of
Humboldt University (Berlin, Germany), an
astrophysicist who became an
astroarchaeologist, writes that Babylonian mathematics, as applied to
astronomy, had
geometrical operations that anticipate modern
calculus.[3-5] He discovered five clay tablets of the period 350-50 BC that explain the motion of
the planet, Jupiter, using a
trapezoidal construction of
velocity data as a
function of
time.[3,5]
Jupiter was a prime object of study in Babylonian astronomy, since Jupiter was identified with their principal
deity,
Marduk (see figure). While pondering the significance of some clay tablets, Ossendrijver was gifted with
photographs of a similar, uncataloged tablet at the British Museum by
Assyriologist,
Hermann Hunger of the
University of Vienna.[4] Ossendrijver was able to connect the trapezoidal calculation of four tablets with Jupiter through this additional tablet.[5]

The Babylonian god, Marduk, as found on a cylindrical seal.
Marduk, who is associated with the planet, Jupiter, is shown on the seal with his pet dragon, sirrush.
Zeus, the principal deity of the ancient Greeks, and Jupiter, the principal deity of the Romans, were also identified with the planet Jupiter.
(Modified Wikimedia Commons image.)
As most of us remember, the trapezoidal method of finding the
area under a
curve was a prelude to
integral calculus. In the Babylonian tablets, such a
construction was used to
interpolate the
position of Jupiter through
ratios of the areas under the velocity
vs time curve. The area under such a curve, velocity
multiplied by time, gives distance. In this astronomical case, the velocity is degrees per period, so the distance is specified as degrees (see figure). Such an operation was first seen in
Europe in the
14th century.[3]
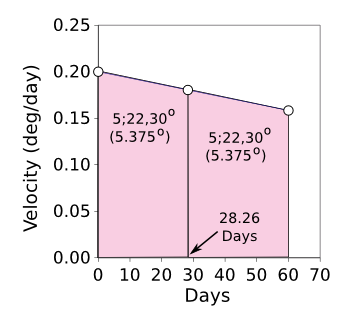
Equal area construction for the motion of Jupiter.
The areas are given in sexagesimal notation and their decimal equivalent.
(Created from data in ref. 3 using Gnumeric and Inkscape.[3]
![]()
References:
- Bertha Morris Parker, "The Golden Book of Science for Boys and Girls (A Giant Golden Book)," Simon and Schuster, January 1, 1956, 97 pp. (via Amazon).
- Irving Adler, "The Giant Golden Book Of Mathematics: Exploring The World Of Numbers And Space," illustrated by Lowell Hess, Golden Press, January, 1960, (via Amazon).
- Mathieu Ossendrijver, "Ancient Babylonian astronomers calculated Jupiter's position from the area under a time-velocity graph," Science, vol. 351, no. 6272 (January 29, 2016), pp. 482-484, DOI: 10.1126/science.aad8085.
- Ron Cowen, "In Depth - Archaeology - Ancient Babylonians took first steps to calculus," Science, vol. 351, no. 6272 (January 29, 2016), p. 435, DOI: 10.1126/science.351.6272.435.
- Michelle Hampson, "Ancient Babylonians Used Advanced Geometry to Track Jupiter," AAAS News, January 27, 2016.
Permanent Link to this article
Linked Keywords: Science; education; child; children; The World of Science; Golden Book of Science; author; woman; gender equality; mathematics; Irving Adler; Western Publishing; Golden Press; New York; illustration; illustrated book; youth; young; scientist; publication; Seven Wonders of the Ancient World; Great Pyramid of Giza; Anno Domini; BC; Hanging Gardens of Babylon; Temple of Artemis at Ephesus; Statue of Zeus at Olympia; Mausoleum at Halicarnassus; Colossus of Rhodes; Lighthouse of Alexandria; King Nebuchadnezzar II; Babylon; Iraq; mythology; mythical; garden; Assyrian; monarch; king; Sennacherib; Nineveh; painting; Ferdinand Knab; gardening; quirk; Babylonian numerals; number system; sexagesimal; radix; base-60 system; Sumer; Sumerian; logic; highly composite number; cooking; cook; ratio; scaling; recipe; wedge-tipped; stylus; fractional; decimal; Wikimedia Commons; degree; circle; hexagon; Babylonian astronomy; astronomer; astronomical observations; clay tablet; Venus tablet of Ammisaduqa; Venus; Halley's comet; decipherment; deciphering; scribble; hen-scratching; student; British Museum; London; Mathieu Ossendrijver; Humboldt University (Berlin, Germany); astrophysics; astrophysicist; archaeoastronomy; astroarchaeologist; astronomy; geometry; geometrical; operation; calculus; the planet, Jupiter; trapezoid; trapezoidal construction; velocity; data; function; time; deity; Marduk; photograph; Assyriologist; Hermann Hunger; University of Vienna; cylinder; cylindrical; emblem; seal; dragon; sirrush; Zeus; ancient Greece; ancient Greeks; Jupiter mythology; Roman Empire; Romans; area; curve; integral calculus; construction; interpolation; interpolate; position; ratio; multiplication; multiply; Europe; 14th century; Gnumeric; Inkscape; Bertha Morris Parker, "The Golden Book of Science for Boys and Girls (A Giant Golden Book)," Simon and Schuster, January 1, 1956, 97 pp.; Irving Adler, "The Giant Golden Book Of Mathematics: Exploring The World Of Numbers And Space," illustrated by Lowell Hess, Golden Press, January, 1960.