Tikalon Blog by Dev Gualtieri

Reverse Sprinkler

April 8, 2024

Eric Sevareid (1912-1992) was a prominent journalist and World War II correspondent who made the transition from print media to television in its early years. Sevareid was hired by Edward R. Murrow (1908-1965) as a member of the CBS News team, and he served as a commentator on the CBS Evening News for thirteen years.

Wikimedia Commons image

I remember one of Sevareid's commentaries on the CBS Evening News with Walter Cronkite (1916-2009). He contrasted photographs of smiling diplomats in the 1970s with the austere photos earlier in the 20th century. He said that this wasn't because the world was a better place in the 1970's, but that statesmen early in the century all had rotten teeth. I was reminded of this during a recent visit to my dentist, at which we also discussed the physics of oral irrigators, a.k.a., Waterpiks.

In that conversation I somehow remembered Bernoulli's principle, named after Swiss physicist, Daniel Bernoulli (1700-1782). According to Bernoulli's principle, the velocity of a fluid increases as the diameter of a pipe decreases. For an incompressible fluid, the fluid velocity v₁ changes to a new velocity v₂ in proportion to the ratio of the pipe cross-sectional areas A₁ and A₂,

v₂ = v₁(A₁/A₂)

My Waterpik is not the only example of hydraulics in my house. We get our water from a well that's 75 feet deep with the water pump at the bottom, rather than in our house. The reason for this is that an ideal pump producing a perfect vacuum can only lift water 33.9 feet. We can't suck the water from the well; so, we need to pump it out.

Piston pumps in the 16th century were only capable of sucking water to a height of about 24 feet. As described in Book VI of De re metallica (On the Nature of Metals) by Georgius Agricola (1494-1555), miners would suck water from their mines using a cascade of pumps to lift water to one level, and then upwards to another. As he writes,

Figure 185 from De Re Metallica by Georgius Agricola (1494-1555)

"Because these pumps can only lift water about 24 feet, batteries of pumps are required for the deepest mines. It is composed of several pumps, which do not, like those last described, go down into the shaft together, but of which one is below the other, for if there are three, as is generally the case, the lower one lifts the water of the sump and pours it out into the first tank; the second pump lifts again from that tank into a second tank, and the third pump lifts it into the drain of the tunnel."[1]

(Project Gutenberg image.[1])

Another common household hydraulic device is the lawn sprinkler. Most of these in use today have a mechanism that sprays water in an overhead arc or in a radial pattern to ensure coverage of a large area. The simplest, but less effective, type of lawn sprinkler is the one that uses the force of water jets to propel a radial spray. This type of lawn sprinkler was described a hundred and fifty years ago in the 1883 physics textbook, The Science of Mechanic by Austrian physicist, Ernst Mach (1838-1916) (see figure).[2] Albert Einstein (1879-1955) was influenced by Mach's concept of inertia. The fundamental idea of this device, using steam instead of water, was described by Greek mathematician and engineer, Hero of Alexandria (fl. 60 AD), in his book, Pneumatica. I wrote about this device, called an Aeolipile, in a previous article (Steam Power, January 28, 2011).

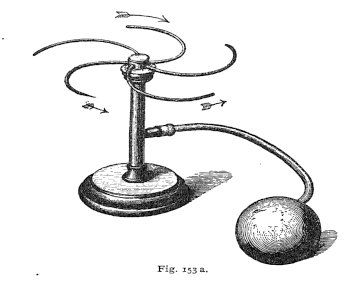
Figure 153a from the Science of Mechanics (1883) by Ernst Mach. This is an air propelled version of a lawn sprinkler.

Mach, who was an accomplished experimental physicist, is famous also for the eponymous theoretical principle known as Mach's principle.

The ancients would reference things to the "fixed stars," and this same idea is behind Mach's principle that a local spinning object has centrifugal force because of its spin relative to all the other matter in the universe.

(Figure from ref. 2.[2] Click for larger image.)

The device in the above figure, an air propelled version of a lawn sprinkler, will rotate when the attached rubber bulb is pressed. Mach noted that when the rubber bulb is released, sucking air, the device showed "no distinct rotation," a clear demonstration of time-reversal asymmetry.[2] Nobel Physics Laureate, Richard Feynmen (1918-1988), became interested in the reverse sprinkler when he was a graduate student at Princeton University, and he mentioned it in his autobiography, Surely You're Joking, Mr. Feynman!.[3] The problem has since been called the Feynman sprinkler. Some experiments using low friction spinners and high external fluid pressures resulting in high inflow rates have shown a reverse spin. However the mechanism that causes the reverse spin was elusive.

As is often the case, one simple experiment is worth more than a hundred theoretical papers. A recent experiment by scientists from New York University (New York, New York) and the Colorado School of Mines (Golden, Colorado) elucidated the mechanism of the reverse sprinkler.[4-7] They used a sprinkler with an ultra-low-friction rotary bearing immersed in water containing dyed microparticles illuminated by a laser to track the fluid flow on high-speed video.[4-7] The reverse sprinkler's rotation was fifty times slower than that of a forward sprinkler, and the reverse motion was the result of an asymmetry in the inward flowing water jets caused by the rotation of the sprinkler, a consequence of the water's being forced outward by centrifugal force.[5-7]

Flow pattern of a reverse sprinkler, as revealed by laser illuminated microparticles. For this visualization, movement of the device was prevented. A complex flow is seen internal to the sprinkler. (Still image from an NYU Applied Mathematics Laboratory video. Click for larger image.)

Previous reverse sprinkler experiments have shown mixed results, some demonstrating steady rotation, some just transient rotation, and others having an oscillating rotation direction.[7] Knowing that the friction forces acting on the sprinkler would greatly affect its rotation, the research team designed a sprinkler head that floats freely when immersed in water.[5-6] Rotational forces that allowed both normal and reverse rotation were achieved by adjusting a connected siphon tube from the center of the sprinkler to a side tank.[5] The transparent sprinkler head allowed observation of details of the water flow both internal and external to the sprinkler.[7]

The experiments found that the reverse flow case is not a time-reversed version of normal flow.[5] The torque required for the reverse rotation arises from an asymmetry in the direction of the inward water jets caused by the rotation itself.[5] The researchers developed a mathematical model of the flow patterns that explains the observations.[4-5] This research was funded by the National Science Foundation.

Reverse Sprinkler

References: