Tikalon Header

Singing Saw

July 4, 2022

In several previous articles, I've mentioned kitchen chemistry, the use of common household materials in doing simple experiments. There's also kitchen music, well known to pot-banging children and their parents, in which kitchen items are used to produce music. Aside from percussive instruments such as pots and pans, there's also the glass harp in which rubbing a finger around the rim of a beverage glass results in a tone that depends on the type of glass and the level of fluid that it contains. The very inventive Benjamin Franklin (1706-1790) created an advanced version of this in his 1761 glass harmonica.

A 1492 illustration by Franchino Gafori depicting a glass harp

A 1492 woodcut by Franchino Gafori depicting a glass harp in Theorica musicae (Milan, 1492).

This image appeared on page 11 of Thomas Bloch's L'harmonica de verre ou glassharmonica: données et synthèse historique, organologique, acoustique et bibliographique sur l'instrument de Benjamin Franklin et sur les instruments dérivés, Bulletin FRBNF35344835 of the Conservatoire National Supérieur de Musique de Paris, 1988-89.

(Portion of a Wikimedia Commons image.)

Just as in rubbing a bow on a violin string, rubbing a finger around the rim of a beverage glass will excite a standing wave in the rubbed medium.[1] In the case of a violin, the medium is the string, the standing wave of the string is along its length, and the frequency is determined by the linear density and tension of the string. For the singing glass, the standing wave is in the body of the glass, extending between the rim and the water line, and that explains why a change in the water level changes the frequency.[1] The sound of the violin string is amplified by the violin body, and the sound of the singing glass is amplified in the air space inside the glass. You can also imagine affixing a singing glass to something like a violin body to produce a higher amplitude sound.

Moving from the kitchen to the cellar workshop leads us to the musical saw. A musical, or singing saw, is a flexible steel hand saw, bent into an "S" shape, that's bowed on its flat edge. The musical saw is used in folk music in the United States and other countries, a likely result of the ubiquity of handsaws on farms. The popularity of the singing saw increased with easy access to inexpensive, flexible steel in the early 19th century.

A musical saw performance is something you might see in a video clip of a 1950s television variety show, such as The Ed Sullivan Show. The actress, Marlene Dietrich (1901-1992) was a notable musical saw artist, a consequence of her violin training. Today's youth have likely never heard of a musical saw, Ed Sullivan, or Marlene Dietrich. On the other hand, Baby Boomers would confuse The Weeknd with the weekend.

The key to the acoustics of the musical saw is its "S" shape, created by holding the saw handle between the knees and bending it into shape with one hand. vibration is damped in the curved parts of the saw blade, but the center of the S-curve is relatively flat, creating a sweet spot for tone production (see figure). Bowing produces standing waves across the blade width; and, as a result, forming this area in a wider section of blade leads to lower frequency sound. Since a width difference of 2:1 is needed to produce an octave's range of frequency, there are saws designed specifically for music production. Adjusting the S-curve allows control of the position of the flat section and the frequency produced.

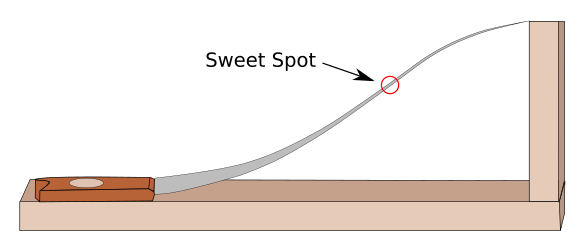
Schematic of a singing saw clamped into an 'S' shape on a test jig.

Schematic diagram of a singing saw clamped into an 'S' shape on a test jig. The center of the flat area, called the "sweet spot," is the place at which bowing produces the best sound. The sweet spot is what physicists would call an area of localized vibrational modes, a confined area which resonates without losing energy at the edges. (Created using Inkscape. Click for larger image.)

There have been scientific studies of the acoustics of the singing saw,[1-3] the most recent of which, by scientists at Harvard University (Boston, Massachusetts), has appeared as an open access article in the Proceedings of the National Academy of Sciences.[3] Musical instruments, such as the aforementioned glass harp, need to produce sustained notes.[3] This quality is a part of the design of musical instruments from tubas to tablas, but a saw is not designed to incorporate an acoustic resonator as would a musical instrument.[3-4]

The resonant area for a singing saw is localized at the inflection point between the two bends of its "S" shape.[3] Says Petur Bryde, a co-first author of the paper describing this study,
"How the singing saw sings is based on a surprising effect... When you strike a flat elastic sheet, such as a sheet of metal, the entire structure vibrates. The energy is quickly lost through the boundary where it is held, resulting in a dull sound that dissipates quickly. The same result is observed if you curve it into a J-shape. But, if you bend the sheet into an S-shape, you can make it vibrate in a very small area, which produces a clear, long-lasting tone."[4]

In their experiments, the research team clamped a saw in two configurations - a "J" shape and an "S" shape. The "S" shape has an inflection point (the sweet spot), while the "J" shape doesn't.[4] It was found that only the "S" shape will sing, and the details of its shape are unimportant.[4] The "S" could have a big curve at the top and a small curve at the bottom, or visa versa.[4] The existence of the inflection point is all that matters.[4]

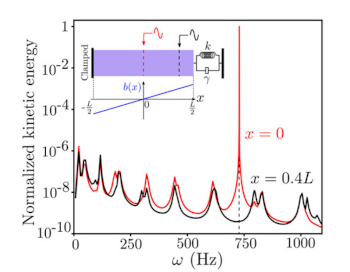
Sweet spot resonance for a singing saw

Sweet spot resonance for a singing saw.

These are resonance curves for a shell with curvature as shown, periodically driven at the inflection point (x = 0, the red line) and away from it (x = 0.4, the black line) for varying frequency, with ω = 740 Hz being the first localized mode.[3]

(Fig. 3a of ref. 3, licensed under the Creative Commons Attribution-NonCommercial-NoDerivatives License 4.0.[3])

Variation in the saw blade curvature along its length controls the localization of trapped acoustic states, and this makes a singing saw the high-quality geometrically tunable oscillator that it is.[3] This has an analogy to the edge states in topological insulators.[3] Topological insulators are electrical insulators that have the surprising property that they will conduct electricity in their surface or edges, but nowhere in their bulk.[4] The opposite curves in a saw produce what are in effect internal edges.[4] The physics behind the singing saw may allow the design of high quality resonators for sensors, nanoelectronics, and photonics.[4] The team plans to expand their research by investigating doubly curved structures, such as bells.[4] This research was funded by the National Science Foundation.[4]

While on the topic of physics as applied to music, I'll mention an interesting study presented at a recent conference and available on arXiv.[6] The study is about rickrolling in the academic literature.[6] Rickrolling is giving a link to an expected topic that's actually a link to the YouTube video of Rick Astley's, "Never Gonna Give You Up."[7] Possibly because of this, the video has more than 1.2 billion views.[7] I was confused the first time I was rickrolled more than a decade ago, but I've come to enjoy both the song and the video. The comment in the arXiv abstract for the study is a rickroll. As of March, 2022, there are 23 academic documents intentionally rickrolling the reader. This is not a huge number, but another indication that scientists and academics are playful people.[7]

References:

  1. Reuben Leatherman, Justin C. Dunlap, and Ralf Widenhorn, "The Fourier Spectrum of a Singing Wine Glass," American Journal of Physics, vol. 87, no. 10 (September 18, 2019), pp. 829-835, doi: 10.1119/1.5124230. A PDF file of this paper appears here.
  2. Randy Worland, "Vibroacoustics of the Musical Saw: Experimental Measurements of Trapped Mode Shapes and Frequencies vs. Blade Curvature," The International Institute of Acoustics and Vibration, ICSV26 (Montreal, Canada, July 7-11, 2019), PDF File.
  3. Suraj Shankara, Petur Brydeb, and L. Mahadevana, "Geometric control of topological dynamics in a singing saw," Proc. Natl. Acad. Sci, vol. 119, no. 17 (April 21, 2022), Article no.e2117241119, https://doi.org/10.1073/pnas.2117241119. This is an open access publication with a PDF file here.
  4. Leah Burrows, "The physics of a singing saw," Harvard University School of Engineering and Applied Sciences Press Release, April 21, 2022.
  5. Austin Blackburn plays 'Ave Maria' on musical saw, YouTube Video by msawman, June 10, 2008.
  6. Benoit Baudry and Martin Monperrus, "Exhaustive Survey of Rickrolling in Academic Literature," arXiv, April 14, 2022. Part of the 2022 SIGBOVIK conference. SIGBOVIK is an annual multidisciplinary conference specializing in lesser-known areas of academic research. The 2022 conference proceedings can be found as a PDF file here.
  7. Rick Astley, "Never Gonna Give You Up (Official Music Video), YouTube, October 25, 2009.