Tikalon Blog by Dev Gualtieri

Fermi-Pasta-Ulam

April 20, 2015

Of all the scientific disciplines, physics is the most mathematical. It's apparent, as Eugene Wigner wrote in 1960, that mathematics is unreasonably effective in describing the world around us.[1] The use of mathematics in the natural sciences can be traced back at least as far as the third century BC, when Greek mathematician , Eratosthenes , used trigonometry to determine the radius of the Earth to within two percent of its established value.

Physicists were quick to use the first computers, including mechanical calculators made by Marchant and Friden. During the Manhattan Project, several wives of the Los Alamos scientists, including Edward Teller's wife, Mici, acted as human computers by doing calculations on such machines.[2] The versatile Richard Feynman acted as a de facto calculator repairman. Feynman teamed for calculator repair with Nicholas Metropolis. Metropolis was instrumental in early physics computing, as I wrote in a previous article (Nicholas Metropolis, June 11, 2010).

As Feynman wrote in his book, Surely You're Joking, Mr. Feynman!, Los Alamos eventually obtained an IBM punched-card computer.[3] This type of computer had proven its worth in calculating planetary orbits, so it was a safe bet. The first task of the Los Alamos IBM computer was a calculation to simulate a shaped-charge implosion. As a test of the efficacy of this new machine, the calculations were done simultaneously by both the ladies on the calculators and the IBM computer. The ladies were able to calculate at the same pace, but the IBM could function continually, without rest, so it won the competition.[3-4]

John von Neumann, who originated the shaped-charge idea, had an early interest in computers, and he arranged to have an unclassified Los Alamos calculation computed on the Harvard University Mark I electromechanical calculator. The Mark I took longer to calculate, but it did the calculation with more precision than the Los Alamos IBM computer.[2] Von Neumann was subsequently involved with ENIAC, the first electronic general-purpose computer, and its successor, the 6,000 vacuum tube EDVAC, one of the first stored-program computers.

Shrinking computer hardware, ENIAC and successors.

Shrinking computer hardware, ENIAC (left) and successors. The smallest circuit board is from the BRLESC-I.

(U.S. Army Photo 163-12-62, via Wikimedia Commons.)

At Los Alamos, Feynman was a pioneer in parallel computing. By interleaving colored punch-cards containing different calculations, Feynman was able to do several computations at one time.[3] After the war, progress in computing continued at Los Alamos, with Metropolis building the MANIAC (mathematical analyzer, numerical integrator and computer) in 1952.[2] The MANIAC was a stored program computer.[4] Software progress was made, also, with von Neumann, Metropolis, and Stanislaw Ulam inventing the Monte Carlo method.[2,5] Ulam, whose uncle enjoyed gambling at the Monte Carlo casino, gave the method its name.[4]

Physicists enjoy their play as much as their work. Feynman would "crack" the safes at Los Alamos while he was there, an adventure simplified by the fact that few safe owners ever changed the default combination.[3] In 1956, Paul Stein and Mark Wells, reportedly with assistance from John Pasta, later head of the department of computer science at the University of Illinois at Urbana-Champaign, created a simplified chess program, called "Los Alamos Chess."[4,6] Because of the limited capability of MANIAC I, the game had a 6x6 chess board, and there were no bishops. The game was good enough to beat at least some humans.[4]

Pasta collaborated in a significant physics simulation with Fermi and Ulam, now called the Fermi-Pasta-Ulam Problem (FPU).[7-10] Physicists are always interested in the behavior of simplified model systems, as illustrated by the particle in a box problem in quantum mechanics. In 1953, Fermi proposed a computer simulation of the temporal evolution of the motion of a vibrating nonlinear string that's initially excited into a single oscillatory mode.[7] This would be one way to simulate how heat is conducted in solids.

Fermi and Ulam expected that the energy of this mode would be transferred to other modes, and that this equilibrium state would happen after a short relaxation time. Instead, after an initial damping of the original mode, it returned to its higher amplitude, a feature of interacting solitons. Of course, everyone blamed some computer error, but they found that the result was real.[7] Fermi died in 1954, but this computer simulation was published in a 1955 Los Alamos technical report. Later, after much longer simulations, it was found that an equilibrium state is finally attained, but the way the system attains this equilibrium was not understood.[8-10]

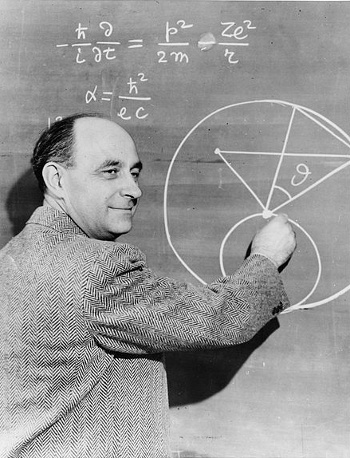Enrico Fermi (1901-1954).

This is my favorite photo of Fermi. He might be smiling because he's just written an incorrect expression for α, the fine-structure constant.

In cgs units, it would be e²/(ħc).

(Smithsonian Institution photograph, via Wikimedia Commons.)

An international team of physicists and mathematicians from the Università degli Studi di Torino and Istituto Nazionale di Fisica Nucleare–Sezione Torino (Turin, Italy), the University of East Anglia (Norwich, United Kingdom), and Rensselaer Polytechnic Institute (Troy, New York) has now explained the eventual emergence of equipartition of energy states in the FPU model system.[8-10] They developed a general approach to such problems, so their method can be applied to other weakly nonlinear dispersive waves.[8]

What Fermi, Pasta, and Ulam had expected from their calculation was that their model system would reach thermal equilibrium, a state in which the system energy would partition itself among all possible modes. When the model ran, it appeared that the energy, stored in just a single mode as an initial condition, was being distributed. However, after subsequent iterations it was found that the energy would periodically be dispersed, but then reconcentrated in the initial mode to about 97% of its value.[9] The development of more powerful computers allowed the simulation to proceed to much longer time scales, and it was found that thermal equilibrium did occur, but by an unknown mechanism.[10]

The model system is a linear chain of 16, 32, or 64 masses connected by a nonlinear quadratic spring.[9] The research team attacked the problem by methods known to work in wave turbulence theory.[10] As it turns out, there's a mixing of modes in the system in which coincidences of modes allows a gradual transfer of energy. When precisely six modes interact, there's an irreversible transfer of energy, but it takes many iterations to transfer enough energy to reach thermal equilibrium.[8-9]

For small-amplitude random waves, such a mode interaction occurs on an extremely long timescale. It was found that the timescale is of the order of 1/ε⁸, where ε is a very small system parameter.[8] The mode mixing does not have a threshold, and the equipartition of energy will happen even for small nonlinearity.[8] Says Yuri Lvov, an author of the paper and a professor of mathematical sciences at Rensselaer Polytechnic Institute,

"My collaborators and I have shown that interactions of triads, quartets, and quintets are reversible; in other words, they do not bring the FPU system closer to thermal equilibrium. However, the interaction of waves in sixtets does lead to irreversible transfer of energy. It takes the cooperation of six different waves to produce an interaction that is irreversible and, because of that, the process is extremely weak and very slow. That is why it takes so long to approach thermal equilibrium for the FPU system."[9]

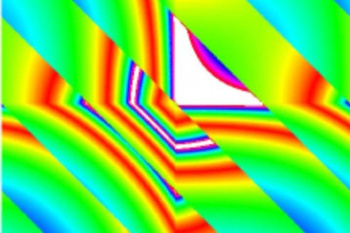Pretty picture.

This is a visualization of the resonance manifold in the Fermi-Pasta-Ulam problem.

(RPI image by Yuri Lvov.)

Fermi-Pasta-Ulam

References: